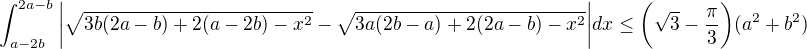Matematické Fórum

Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.

Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.

Nejste přihlášen(a). Přihlásit

Stránky: 1

 

#1 29. 04. 2019 15:20 — Editoval stuart clark (29. 04. 2019 15:21)

stuart clark
Příspěvky: 1015
Reputace:   7 
 

Integration Inequality

$\displaystyle \int^{2a-b}_{a-2b}\bigg|\sqrt{3b(2a-b)+2(a-2b)-x^2}-\sqrt{3a(2b-a)+2(2a-b)-x^2}\bigg|dx\leq \bigg(\sqrt{3}-\frac{\pi}{3}\bigg)(a^2+b^2)$

Offline

 

#2 30. 04. 2019 02:25 — Editoval laszky (30. 04. 2019 02:29)

laszky
Příspěvky: 2376
Škola: MFF UK, FJFI CVUT
Reputace:   197 
 

Re: Integration Inequality

↑ stuart clark:

Hi. Is it correct that there can be square roots of negative values? For instance, for $a=2$, $b=1$ we obtain

$\displaystyle \int^{3}_{0}\bigg|\sqrt{9-x^2}-\sqrt{6-x^2}\bigg|\,\mathrm{d}x \;\; \leq \;\; \bigg(\sqrt{3}-\frac{\pi}{3}\bigg)\cdot5$

Hence, absolute value of complex number has to be considered for 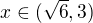$x\in(\sqrt{6},3)$.

Offline

 

#3 30. 04. 2019 16:39

stuart clark
Příspěvky: 1015
Reputace:   7 
 

Re: Integration Inequality

I will conform it from original source ↑ laszky:

Offline

 

#4 08. 05. 2019 12:58

stuart clark
Příspěvky: 1015
Reputace:   7 
 

Re: Integration Inequality

//forum.matweb.cz/upload3/img/2019-05/13071_20190502_135153.jpg

Offline

 

#5 18. 05. 2019 16:36

laszky
Příspěvky: 2376
Škola: MFF UK, FJFI CVUT
Reputace:   197 
 

Re: Integration Inequality

↑ stuart clark:

Hi, the expressions under the square root are not the same as you have written.

Offline

 

#6 21. 05. 2019 05:29

stuart clark
Příspěvky: 1015
Reputace:   7 
 

Re: Integration Inequality

Yes ↑ laszky: you are Right

Offline

 

 

Stránky: 1

Zápatí

Powered by PunBB
© Copyright 2002–2005 Rickard Andersson