Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#1 23. 06. 2012 17:06
tan(x)=x
Pozdravujem.
Tu je jeden pekny problem:
Nech  su vsetky pozitivne korene rovnice
su vsetky pozitivne korene rovnice  uporiadane stupajuco.
uporiadane stupajuco.
Dokazte, ze  .
.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#3 23. 06. 2012 21:56
Re: tan(x)=x
Ahoj ↑ pietro:,
Je viac dokazov tejto vlasnosti, no ale bol by som rad keby to niekto skusil najst.
Mas pravdu, tento problem je v niecom fascinujuci.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#4 24. 06. 2012 00:05
- check_drummer

- Příspěvky: 4904
- Reputace: 105
Re: tan(x)=x
↑ vanok:
Ahoj, zkoušel jsem řešit přes Taylorův rozvoj, ale ten je díky nespojitosti tan(x) definován jen na intervalech  , na čemž jsem skončil. Rovněž jsem zkoušel spočítat pro uvedená
, na čemž jsem skončil. Rovněž jsem zkoušel spočítat pro uvedená 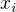 hodnotu
hodnotu  , ale zatím také nic...
, ale zatím také nic...
"Máte úhel beta." "No to nemám."
Offline
#5 24. 06. 2012 13:25
Re: tan(x)=x
↑ check_drummer:,
Pozdravujem, jedna moznost na dokaz je pouzitie theoremy o residuach (Théorème des résidus,Residue theorem) aplikovanu na dobre vybranu funkciu.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#6 30. 06. 2012 12:41
Re: tan(x)=x
Indikacia:
Ak chce niekto riesit tento problem, vdaka teoreme o residuach, tam moze pouzit tuto funkciu , ktorej residus su
, ktorej residus su 
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#7 09. 07. 2012 15:42
Re: tan(x)=x
Pozdravujem vsetkych, co zaujal tento problem.
Tu je dokument, kde je krasne dokazany dany problem viacerymy metodamy, ako aj ine vlasnosti, tykajuce sa tohto problemu
http://alain.pichereau.pagesperso-orang … %29=x.html
Sice je to po francuzky, ale maly slovnik postaci :-)
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline




