Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#1 11. 04. 2016 04:38
- stuart clark

- Příspěvky: 1015
- Reputace: 7
Probability
There is a line of length  unit (where
unit (where  is fixed positive real number), The point
is fixed positive real number), The point  and
and  choosen randomly
choosen randomly
(uniformly) on this line, Let  be a fixed real number satisfying
be a fixed real number satisfying 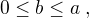 Then probability that
Then probability that 
Offline
#2 12. 04. 2016 00:52 — Editoval vanok (12. 04. 2016 08:41)
Re: Probability
Hi ↑ stuart clark:,
Let us choose the coordinates of P, Q x and y respectively. So  expresses as
expresses as  and so I(x,y) bas to be in 2 regions of squared ABCD of coordinates (0,0), (a , 0), (a , a), (0, a) in an orthonormal Cartesian coordinate system, containing vertices B and D and bounded by two straight lignes of equations
and so I(x,y) bas to be in 2 regions of squared ABCD of coordinates (0,0), (a , 0), (a , a), (0, a) in an orthonormal Cartesian coordinate system, containing vertices B and D and bounded by two straight lignes of equations 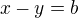 and
and 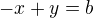 . (Make the figure)
. (Make the figure)
So your probability is 
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline