Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#226 13. 02. 2016 12:54
Re: najkrajsia teorema
Pozdravujem,
Ak hladate pre poucenie nejake blogy, tak mozte zacat tu
http://blogs.ams.org/blogonmathblogs/#s … 2fe3r.dpbs
Iste najdete nieco pekne, krasne a aj najkrajsie.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#227 14. 02. 2016 19:49
Re: najkrajsia teorema
Pozdravujem
Tu https://hcmop.wordpress.com/2012/03/21/ … x-numbers/
je blog kde najdete pekne citanie o identite od Sophie Germain.
Aj v 18 storoci boli tiez matematicky.
Inac som pisal dnes o tej identite na stredoskolskom fore.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#228 01. 03. 2016 12:10
Re: najkrajsia teorema
Nechť  jsou celá čísla, potom počet kroků při výpočtu největšího společného dělitele Euklidovým algoritmem nepřesáhne
jsou celá čísla, potom počet kroků při výpočtu největšího společného dělitele Euklidovým algoritmem nepřesáhne  ,
,
kde  je zlatý řez,
je zlatý řez,
přitom toto maximum nastane po sobě jdoucí Fibonacciho čísla.
Příspěvky psané červenou barvou jsou moderátorské, šedá je offtopic.
Offline
#229 09. 03. 2016 12:43 — Editoval vanok (09. 03. 2016 19:58)
Re: najkrajsia teorema
Pozdravujem
http://www.dankalman.net/AUhome/writingawards.html
Vyssie najdete vela zaujimavych citani.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#230 11. 03. 2016 09:35
#231 18. 03. 2016 20:33
Re: najkrajsia teorema
Pozdravujem.
Novy rekord
http://www.mersenne.org
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#232 20. 03. 2016 22:05
- check_drummer

- Příspěvky: 4897
- Reputace: 105
Re: najkrajsia teorema
↑ byk7:
Ahoj, čistě formálně to není zobecnění, protože Tvé tvrzení pro 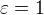 nepožaduje
nepožaduje  , ale Bertrandův postulát ano.
, ale Bertrandův postulát ano.
"Máte úhel beta." "No to nemám."
Offline
#233 01. 04. 2016 23:24
Re: najkrajsia teorema
Pozdravujem
Zaujimave citanie
http://www.math.lsa.umich.edu/~krasny/m … -Kline.pdf
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#234 06. 04. 2016 13:31
Re: najkrajsia teorema
Pozdravujem,
Dalsi pekny blok
http://experimentalmath.info/blog/
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#235 08. 04. 2016 21:32 — Editoval vanok (19. 05. 2016 17:08)
Re: najkrajsia teorema
Pozdravujem,
tu pisem skor o peknom dokaze, o ktorom som uz tu na fore pisal.
Ide o dokaz nerovnosti Cauchy-Schwarz,
Pre  nenulove, mame
nenulove, mame .
.
Rovnost plati len a len ak 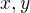 su linearne zavisle.
su linearne zavisle.
A teraz pozrite na tento nadherny dokaz: , je KLADNE... a tym je dokaz na 99% ukonceny.
, je KLADNE... a tym je dokaz na 99% ukonceny.
Edit.
Upresnenie.
E je euklidovsky priestor... Pripominam, ze skalarny sucin je symetricka definitna pozitivna bilinearna forma na realnom vektorovom priestore.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#236 09. 04. 2016 12:54 — Editoval vanok (09. 04. 2016 12:54)
Re: najkrajsia teorema
Pridam aj to posledne 1%.
A pochopitelne 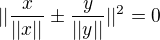
len a len ak 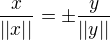
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#237 17. 05. 2016 07:56 — Editoval vanok (17. 05. 2016 08:31)
Re: najkrajsia teorema
Pozdravujem
Prijemne citanie
http://www.purplemath.com/modules/index.htm
A tiez zaujimave citanie o NF
http://plato.stanford.edu/entries/quine-nf/
... Ide o jednu teoriu mnozin o ktorej ste asi nepoculi.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#238 19. 05. 2016 13:28 — Editoval vanok (19. 05. 2016 16:56)
Re: najkrajsia teorema
Pozdravujem
Este doplnok k #236
http://www.uni-miskolc.hu/~matsefi/Octo … le1_19.pdf
Na jednom blogu som nasiel este inu rovnost
Da sa pouzit tiez na dokaz CS....
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#239 26. 06. 2016 12:37
Re: najkrajsia teorema
Blog kde je vela krasneho ci najkrajsieho
https://usamo.wordpress.com/
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#240 28. 06. 2016 17:18
Re: najkrajsia teorema
Toto http://www.mathcounterexamples.net sa mi celkom paci.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#241 09. 07. 2016 13:52
Re: najkrajsia teorema
Toto moze byt uzitocne
http://webspace.ship.edu/msrenault/divi … Tricks.pdf
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#242 13. 07. 2016 11:20
Re: najkrajsia teorema
Zaujimava, uzitocna adressa https://oeis.org kde sa najde vela najkrajsieho o postupnostiach z celymi hodnotami.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#243 20. 07. 2016 23:28 — Editoval vanok (20. 07. 2016 23:29)
Re: najkrajsia teorema
Pozdravujem,
Tu http://www.math.sjsu.edu/~hsu/pdf/sequences.pdf si mozte prectat clanok, "Niektore velmi zaujimave postupnosti".
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#244 21. 07. 2016 10:11
Re: najkrajsia teorema
Pozdravujem,
poucne citanie
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#245 31. 07. 2016 16:36
Re: najkrajsia teorema
Pozdravujem
Toto URL https://www.quora.com , vam pomoze nast aj zaujimave informacie tykajuce sa matematiky.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#246 12. 08. 2016 10:48
Re: najkrajsia teorema
Pozdravujem,
Poznate toto URL. ?
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#247 15. 08. 2016 13:38 — Editoval vanok (15. 08. 2016 14:00)
Re: najkrajsia teorema
Pozdravujem.
Tu najdete kriterium delitelnosti cislom 7.
Skuste
Dokazete ho dokazat?
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#248 25. 08. 2016 14:08
Re: najkrajsia teorema
Pozdravujem,
Tu http://www.pims.math.ca/resources/publications/pi-sky sa pise o casopis pre high-schole. Iste vas to pobavi, ak vas zaujima matematika.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#249 28. 08. 2016 15:55
Re: najkrajsia teorema
Pozdravujem,
Tu http://unsolvedproblems.org najdete toho vela zaujimaveho.
Aj vela prekvapeni.
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#250 18. 09. 2016 23:10
Re: najkrajsia teorema
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline

