Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#1 15. 03. 2019 07:40
- stuart clark

- Příspěvky: 1015
- Reputace: 7
matrix equation
Number of solution of matrix equation 
Offline
- (téma jako vyřešené označil(a) byk7)
#5 15. 03. 2019 11:24
Re: matrix equation
Hi ↑ stuart clark:,
You can also use that your matrix is diagonalizable
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#6 15. 03. 2019 11:59
#7 15. 03. 2019 12:46
Re: matrix equation
Eigenvalue of 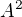 are
are  and
and  .
.
Also 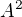 is diagonalizable to
is diagonalizable to .
.
And  .
.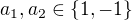 .
.
Remark.  with
with 
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline
#8 16. 03. 2019 16:00
- stuart clark

- Příspěvky: 1015
- Reputace: 7
Re: matrix equation
Thanks ↑ vanok: please explain me I did not understand from 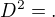 onward
onward
Offline
#9 17. 03. 2019 13:39 — Editoval vanok (17. 03. 2019 13:42)
Re: matrix equation
Hi ↑ stuart clark:,
You can verify that  .
.
( 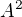 and
and 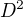 are similar)
are similar)
Srdecne Vanok
The respect, the politeness are essential qualities...and also the willingness.
Do not judge the other one.
Ak odpovedam na nejaku otazku. MOJ PRINCIP NIE JE DAT ODPOVED ALE UKAZAT AKO SA K ODPOVEDI DOSTAT
Offline


