Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#1 15. 02. 2020 15:48
- stuart clark

- Příspěvky: 1015
- Reputace: 7
roots of equation
If  where
where 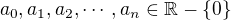 and
and  Then number of real roots of
Then number of real roots of  in
in 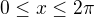
Offline
![kopírovat do textarea $\cos{(mx)} = \cos{[m(2\pi - x)]}$](/mathtex/7a/7ae16a40a3a3a564d277d76c5f203282.gif) for
for ![kopírovat do textarea $x\in [0,\pi]$](/mathtex/96/96c58ab913f49e0415f17388d6692778.gif) and
and 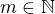 with nonzero
with nonzero![kopírovat do textarea $[0,\pi]$](/mathtex/13/1337ed550e0d119c374eaa7a1ed6fc47.gif) and then double the count.
and then double the count. 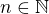 assuming
assuming  , for every
, for every  , there is for
, there is for 

 the sign of the value of
the sign of the value of 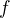 is determined by expression
is determined by expression 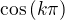 ,
,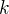 and negative for odd
and negative for odd 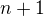 points.
points.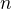 real zeros inside the interval
real zeros inside the interval  can be transformed into
can be transformed into  using transformation
using transformation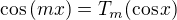 on each term , where
on each term , where  is
is  .
.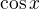 is injective function.
is injective function. .
.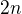 real roots on the interval
real roots on the interval ![kopírovat do textarea $[0,2\pi]$](/mathtex/15/15c80fb5b47715783a5fa5e1aeed97f1.gif) .
.