Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
Stránky: 1
- Hlavní strana
- » Zajímavé úlohy z matematické analýzy
- » exponential equation (TOTO TÉMA JE VYŘEŠENÉ)
#1 22. 01. 2015 09:34
- stuart clark

- Příspěvky: 1015
- Reputace: 7
exponential equation
No. of real solution of the equation 
Offline
- (téma jako vyřešené označil(a) byk7)
#2 22. 01. 2015 19:33
#3 30. 01. 2015 13:31 — Editoval Marian (30. 01. 2015 13:34)
Re: exponential equation
↑ stuart clark:
There is only one real solution. I found the following algebraic approach....
Offline
#5 15. 02. 2015 14:34 — Editoval Pavel (15. 02. 2015 14:36)
Re: exponential equation
↑ stuart clark:
I have another solution that uses a special property concerning of six positive real numbers, see here. (The problem was solved sooner.)
If 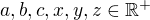 such that
such that
1.  ,
,
2.  ,
,
3. 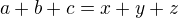 ,
,
4. 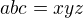
then  ,
,  ,
,  .
.
---
It is clear that 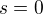 is a solution of the equation
is a solution of the equation 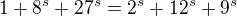 . We show that there is the only solution.
. We show that there is the only solution.
Let  and
and  ,
,  ,
,  ,
, 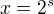 ,
,  and
and  . All the four properties above are satisfied.
. All the four properties above are satisfied.
Then 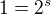 ,
, 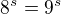 and
and  . However, the equations have no solution.
. However, the equations have no solution.
We can deduce the same conlusion, if  and
and 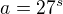 ,
,  ,
,  ,
, 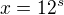 ,
,  and
and  .
.
Backslash je v TeXu tak důležitý jako nekonečno při dělení nulou v tělesech charakteristiky 0.
Offline
Stránky: 1
- Hlavní strana
- » Zajímavé úlohy z matematické analýzy
- » exponential equation (TOTO TÉMA JE VYŘEŠENÉ)
 ,
,  , it is possible to write
, it is possible to write  in a more conveniet way:
in a more conveniet way: and
and  . Further, the manipulations of
. Further, the manipulations of  , we see immediatelly that
, we see immediatelly that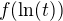 are non-negative. Moreover, they vanish iff
are non-negative. Moreover, they vanish iff  . This implies the desired statement, i.e., the only real zero of
. This implies the desired statement, i.e., the only real zero of  (which corresponds with the choice
(which corresponds with the choice