Matematické Fórum
Nevíte-li si rady s jakýmkoliv matematickým problémem, toto místo je pro vás jako dělané.
Nástěnka
❗22. 8. 2021 (L) Přecházíme zpět na doménu forum.matweb.cz!
❗04.11.2016 (Jel.) Čtete, prosím, před vložení dotazu, děkuji!
❗23.10.2013 (Jel.) Zkuste před zadáním dotazu použít některý z online-nástrojů, konzultovat použití můžete v sekci CAS.
Nejste přihlášen(a). Přihlásit
#2 22. 12. 2018 12:07
Re: ratio of limits
Hi ↑ stuart clark:,
we can use a substitution to remove the n-dependence of the integrands and, with the fundamental theorem of calculus in mind, do some algebraic manipulation: .
.
Since the integrands are left-continuous in 1, we can apply the fundamental theorem of calculus to obtain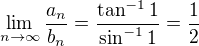 .
.
Offline

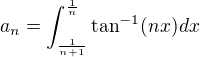 and
and 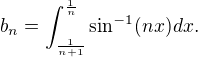 Then
Then