Matematické Fórum

stuart clark · 09. 03. 2019 09:25

Let $g(x)$ is defined for $x>-1$ and has a continuous derivatives. If $g(x)$ satisfies $g(0)=1,g'(0)=0$ and

$(1+g(x))g''(x)=1+x.$ Then maximum value of $g'(2)$ is

and also proving $x=0,g(x)$ has minima and function $g(x)$ is concave upwards

laszky · 17. 03. 2019 12:50

↑ stuart clark:

Hi, numerical solution gives $g'(2)\approx1.594$

Bati · 17. 03. 2019 16:03

Hi ↑ laszky:,
the numerical result is useless unless you provide an error estimate and the method that you used.

laszky · 17. 03. 2019 16:32

↑ Bati:

You have probably never seen the weather forcast :D Is it useless?

jardofpr · 17. 03. 2019 16:45

hey guys,

to follow the comment from ↑ Bati:,

I would add that I believe that also proving global uniqueness for solution on interval $(-1,\infty)$

is necessary to give the numerical solution some weight, assuming some numerical method for ODE was used

in case of ODE with more solutions, numerical approximation of one of the solutions
can be not anywhere close to full picture

(convexity and minimum of the function is very easy in this problem,
I'm just getting curious about the requested maximum estimate)

Bati · 17. 03. 2019 18:29

↑ laszky:
So you basically say that your result is as reliable as a weather forecast... and yes, a bad forecast is pretty useless

↑ jardofpr:
Good point

stuart clark · 23. 03. 2019 13:18

↑ jardofpr:

How can i find convexity and minimum of function. Thanks

jardofpr · 23. 03. 2019 17:03

hi ↑ stuart clark:

There is 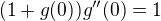$(1+g(0))g''(0) = 1$ therefore $g''(0) = \frac{1}{2}$

together with $g'(0)=0$ it says that the function has at least local minimum in $x=0$ .

Furthermore 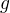$g$ is defined for $x>-1$ so there is $(1+g(x))g''(x)>0$ on the domain of the function

(note that inequality is sharp, never equality)

so in each 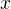$x$ expressions $(1+g(x))$ and $g''(x)$ are either both strictly positive or both strictly negative.

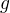As $g''(0)>0$ and given $g$ and its derivatives are continuous, $g''(x)$ stays always positive

and therefore 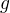$g$ is concave upwards = convex.

This fact also says that $g'(x)$ is strictly increasing, and together with $g'(0)=0$ this gives

$g'(0)<0\,,\,\forall x\in(-1,0)$ and $g'(0)>0\,,\,\forall x\in (0,\infty)$ , which makes the minimum in $x=0$ global.

To the last unanswered question I was yet able to find out that $g'(2) < 2$ by analytical approach
but this is for sure not maximum as it cannot be reached.